Math @ Home
Everyday Math
Day 1: Roman Arch Bridges
Grab all the cushions, books or Jenga blocks at home and try to build an arch bridge.
The forces of a Roman arch so strong that arches can stand without any glue or other adhesive holding them together. Try it for yourself!
How it works:
Its semicircular structure elegantly distributes compression through its entire form and diverts weight onto its two legs, the components of the bridge that directly take on pressure.




Image attributions:
https://www.ancient.eu/image/4407/roman-bridge-ponte-da-vila-formosa-portugal/
https://www.thisiscarpentry.com/2012/01/06/circular-based-arches-part-1/
https://www.thelistlab.net/blog/how-to-make-a-book-arch
Resources:
https://kids.nationalgeographic.com/explore/books/make-this/roman-ice-arch/
Day 2: Leonardo Da Vinci’s Famous Self-Supporting Bridge
Do you have popsicles at home? I did not try with toothpicks or q-tips, but I think that they may also work. Other than those, “Patience” will be the main thing you will need.
Leonardo Da Vinci’s Self-Supporting Bridge is also known as the emergency bridge. No nails, screws, rope, glues, notches, or other fasteners are holding the bridge in place.
You can also watch the step by step instruction video but first I suggest you try by looking at the image below.
How it works:
You will be weaving the sticks together so that the tension between the sticks keeps the bridge together and lifts it off of the ground.
You may also watch the video on YouTube how a father and son build the bridge at their backyards to motivate yourself to keep going :)




Day 3: Cylindrical Mirror and Anamorphic Art
The original is the usage of mirroring paper, but nowadays unwrinkled aluminum foil can be used as well
(But because the images are fuzzier, the observations may not be as clear.)
And a soda or coke can, or any cylindrical object that you can cover with the aluminum foil is ok.
After you create the cylindrical mirror, you may either color an already distorted image (1) or print the polar grid (2) below and create your own anamorphic art.
How it works:
Making anamorphic drawings involves mechanically distorting an image by transferring the image from the square grid (the original image) onto a polar grid (distorted grid.
It is a mapping, or a correspondence, between a cartesian set of coordinates, and a polar set of coordinates.
Place your cylindrical mirror on the circle and look into the mirror to see the image restored.

Resources:
https://anamorphicart.wordpress.com/2010/04/21/cylindrical-mirror-anamorphoses/
https://raft.net/wp-content/uploads/2019/03/278-Anamorphic-Art.pdf
Coloring a Distorted Image
(1) You may color the distorted image below from makezine.com, in that case, my sure that the cylindrical shape you will find at home matches the circle at. The center of the paper.
Polar Grid Template
(2) You can also use this polar grid by printing to make your own drawings;
Day 4: Pi at home
You can do lots of different pi activities at home. I want to list a few very popular ones.
Pi – skyline:
All you need is paper, ruler and crayons, create black bars at the lengths of the digits of Pi and create the skyline for Pi-York, Pi-ris, Pi-lan, Pi-chester ….
Building Pi-City with Lego:
Instead of coloring the digits of Pi, you can use the lego pieces to actually build your Pi-city
Pi – bracelet:
If you have the toolkit, all you need is to give each color a number like; 1-pink, 2-blue, 3-green, 4-red .. And you can start forming your pi bracelet.
Pi Art in a Circle:
Simply divide a circle into 10 equal intervals label them from 0 to 9 if possible each with different colors. Start drawing lines from 3 to 1, 1 to 4, 4 to 1 and go on … Use the same color for the segment with your starting point for each of the drawings …
Pi – Dart Game
If You have Dart Board at home by throwing dart, you can calculate Pi.
Here all you can do is watching this video.
Before I forget, Pi number;
3.1415926535 8979323846 2643383279 5028841971 6939937510 5820974944 5923078164 0628620899 8628034825 3421170679 ...
Image Attributions and for more information please visit;
https://www.whatdowedoallday.com/
http://www.pinkstripeysocks.com/2014/03/pi-day-activity-make-pi-day-bracelets.html




Day 5: String Art
Another paper, pencil and ruler only activity.
But this is to create your art on the paper. If you want to create some 3d art, you can always use a corkboard, pins, and some string.
Even if you have the necessary materials for 3d art, I recommend you start with paper and pencil first.
Draw a big “L” shape on a paper and mark the numbers with equal intervals till 15. Or you can use the templates below. Again, all you are gonna do is drawing straight lines with a ruler to connect the points such as the;
The first point on y-axis goes to the last point on x-axis
The second point on y-axis goes to the second-last point on x-axis …
Spoiler Alert: When you have finished you’ll see that you have created a curve by using straight lines.
You can extend your initial drawing by converting your L shape to a “+” plus sign
Then you can try a 60 angle “<” as your initial figure and complete it to a hexagon by connecting 6 of them from their corners. (Here you can use less number of points on the lines..)
String art is a topic with no limits if you feel like you are interested, make sure you’ll make an internet search.
Have math fun...





Links for the String Art Templates:
Day 6: Vedic Worms
Fill in the multiplication table grid and reduce double-digit numbers to a single digit by adding the digit of the products.
Example: If 9×9=81, add the numbers in the sum (8+1), and put the sum of 9 in the square. If the new sum is also double-digit, add those numbers. Example: 7×8=56; 5+6=11; 1+1=2. Place the number 2 in that square.
We are going to use this number sequences to create the Vedic Worms which are also spirolaterals
Spirolaterals are geometrical figures formed by the repetition of a simple rule. The pattern is formed by drawing line segments of a certain length from a number sequence with a fixed angle and a direction.
Although the spirolaterals can be created with any number sequence, we will use the Vedic Squares we have created. That’s why they are also called “VEDIC WORMS”.
-
Start with a row of numbers you choose. (1,2,3,4,5,6,7,8,9) These numbers will determine the length of each ‘step’ of the ‘spirolateral’.
2. Choose a direction; clockwise (CW) or counterclockwise (CCW)
3. Choose a grid type to draw on (In fact here, you are choosing the angle of your movement)
-
Square Grid (90 degrees )
-
Isometric Grid (60 degrees )
-
Hexagonal Grid (120 degrees ) …
4. Now start drawing spirals through your list.
For example, if we choose CW direction on a square grid with the first row of numbers,
It means 1 step up, 2 steps right, 3 steps down and 4 steps left then repeat like 5 steps up, 6 steps right, 7 steps down and 8 steps left and 9 steps up to complete your drawing.
Please check the post about the Vedic Squares, Worms and The Spirolaterals for the necessary materials..



Day 7: Two (Hinged) Mirrors and Shapes
If you have two small mirrors at home, it means you are ready for this activity.
I had two hinged mirrors that I got from Amazon recently, but any mirrors like some of the foldable vanity mirrors or small Ikea ones will do.
In addition to the pair of mirrors, any shapes, tangram pieces, lego pieces, different shaped toys can be used for this activity.

1. Angles, Reflection, Tessellation:
Tessellation is covering a surface with a shape(s) without any gaps or overlaps.
You can create your tessellation by using lego pieces, shapes anything you can create your design.
Then, use the mirrors to enlarge your design:

Arrange the mirrors as a straight line (180)
to double your design!

Arrange the mirrors with a 120-degree angle in between to triple your design!

Arrange the mirrors with a 90-degree angle in between to ? your design!

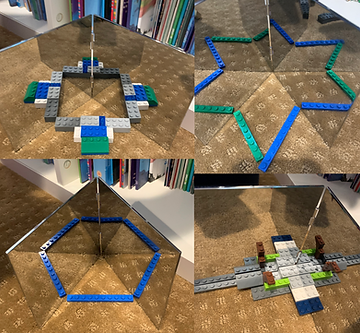

Arrange the mirrors with a 60-degree angle in between to ? your design!
2. Lego Pieces, Other Half, Polygons
Create a car, a space ship, a dragon whatever you like but only half of it, then use the mirror the create the other half. You can do the same by holding the mirrors with different angles to enlarge your designs.
Now use a thin, long lego piece, or any toy that you can use as a line segment.
Arrange your mirrors with a 120 degrees, put the lego piece in between, what is the name of the polygon you have created?

Try the other angles (You can measure the angles with a protractor) what kind of polygons you can create?
What if you want to form a polygon with 12 sides (dodecagon), how are you going to arrange the mirrors?
You can repeat the same activity by drawing a line segment on a paper and putting the mirrors on it by creating different angles between them.

3. Fractions and Creativity
Let's try something else, if you have two identical triangles like tangram pieces ( if don't simply draw, color and cut two identical triangles from paper)
arrange them in all the possible ways to create a square by using the mirrors?
Which angle you need to use to create the square?
How many different designs can you make?
What fraction of your design is purple?
What about your initial shape?
Are those fractions equal?
How many different ways you can divide a square into halves?
If you want to create a snowflake with a shortcut, what would be the angles between your mirrors?
If you want to draw an octopus by drawing only one of its arms, then which angle you need to use?


BY USING TWO MIRRORS, YOU CAN LEARN ABOUT;
-
ANGLES
-
POLYGONS
-
TESSELLATION
-
SYMMETRY
-
REFLECTION
-
FRACTIONS ..


